Since 1998, South Africa has embarked upon a mission that calls upon Curriculum Planners and Implementers to incorporate Indigenous Knowledge Systems (IKS) aspects into the various learning areas. This call is made in the interests of improving teaching and learning for all learners in the country. In this piece, I provide an introduction to the world of IKS and ethnomathematics and reflect on the importance of integrating these systems into the mainstream curriculum.

There are many definitions of IKS, however at the simplest level it can be defined as local knowledge which is unique to a particular culture or society. Ethnomathematics (the relationship between culture and mathematics) bears a very close resemblance to IKS in terms of the rich history and heritage of indigenous and local communities. On the basis of the close resemblance and emphasis, ethnomathematical studies can be considered as one of the components of IKS. The Curriculum and Assessment Policy Statement (CAPS) for mathematics for the different phases identifies a number of Principles which are intended to guide and direct interaction and learning in mathematics classrooms. One of these Principles is:

‘Valuing Indigenous Knowledge Systems [through] acknowledging the rich history and heritage of this country as important contributors to nurturing the values contained in the Constitution’ (DBE, 2011: 5).

This Principle calls upon mathematics educators to have an understanding of IKS broadly and how it can be interpreted and enacted to guide classroom interactions. The implication is that in order for mathematics educators to make real the Principle of valuing IKS as specified in the CAPS, there has to be a consideration of and integration of ethnomathematical studies into the various content areas. Without this effort, this Principle will not be made meaningful in classroom interaction and activities.

Some Definitions of Ethnomathematics and their Implication for the Teaching and Learning of Mathematics

The definitions of ethnomathematics given above suggest that mathematical concepts and processes would be easier and better understood by the learner when they are related to socio-cultural contexts as well as real-life situations. This is likely to make the subject more accessible to most learners as they can relate it to many of the activities and experiences outside the classroom. When this happens, mathematics reduces from being an abstract subject that has no connection to what the learners experience in a real life situation to a subject whose content finds application and relevance to socio-cultural experiences.

Ethnomathematical Studies and the Connection to Mathematical Content

Many ethnomathematical studies have focused upon analysis of various indigenous activities to reveal mathematical concepts and principles that are associated with such activities. Gerdes (1995:8) has identified that ethnomathematical studies revolve around two forms of analysis:

• mathematical traditions that survived colonization and mathematical activities in people’s daily life and ways to incorporate them into the curriculum, and
• culture elements that may serve as a starting point for doing and elaborating mathematics in and outside school.

In both forms of analysis, researchers use their mathematical understanding to interpret an indigenous activity and reveal a variety of mathematical concepts associated with the activity.

In the book ‘Geometry from Africa: Mathematical and Educational Explorations’ Gerdes (1999) illustrates further how mathematical analysis of various indigenous activities may be done. The authors developed a complementary methodology that enabled them to uncover, in traditional, material culture, some implicit geometrical thinking. They looked to the geometrical forms and patterns of traditional objects like baskets, mats, pots, houses, fish traps, and so forth and posed the question: Why do these material products possess the form they have? The answer was that the form of these objects is almost never arbitrary, but generally represents many practical advantages and is, mostly, the only possible or optimal solution of a production problem. The traditional form reflects accumulated experience and wisdom. It constitutes not only biological and physical knowledge about the materials used, but also mathematical knowledge, knowledge about the properties and relations of circles, angles, rectangles, squares, regular pentagons and hexagons, cones, cylinders, and so forth.

Example of Mathematical Analysis based on String Figure Games

Many of us have, during out childhoods, played a string figure game where a loop of string is manipulated using one’s fingers, hands and wrists. The popular “cup and saucer” is depicted below. There are numerous other, vastly more complicated string figures such as the “string gate”.

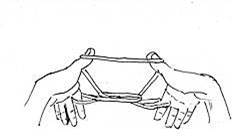
 Cup and saucer  

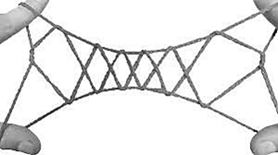
 String gate

The analysis of the string figures reveals a number of mathematical concepts. It is important to indicate that the list of mathematical concepts specified below is not exhaustive. It is possible that other mathematical concepts may be found through further analysis or literature on string figures. The following mathematical concepts were found in the analysis:

(i) Identification of a variety of geometric figures after making the different string figures: triangles; quadrilaterals (depending on how the string was stretched, quadrilaterals also specified into squares and rectangles);

(ii) Specification of relationships between various figures and generalisations drawn from these relationships;

• triangles and quadrilaterals: y = 2x + 2
• quadrilaterals and intersecting points: y = 3x + 1;
• quadrilaterals and the number of spaces (spaces is given by the combination of triangles and quadrilaterals): y = 3x + 2

(iii) Symmetry in terms of performance of some steps in making the figures and disentangling the string along a specific line of symmetry which ensures that the string does not get entangled.

Concluding Thoughts

The integration of ethnomathematical approaches and studies in the teaching and learning of mathematics is almost certainly bound to change how learners view and understand mathematics. It requires educators and curriculum implementers to understand the cultural capital which students possess. This capital is derived from their communities which contain rich cultural and cognitive resources. Teachers should be made aware that these resources can be utilised in the classroom in order to provide culturally appropriate and meaningful mathematics lessons that tap students’ existing knowledge base. It would certainly provide a different form of interpretation of mathematical content such as Euclidean Geometry and Trigonometry.

Author: Professor Mogege Mosimege (Chief Research Specialist) 

With contribution from Dr Andrea Juan (Post Doc Fellow)

Education and Skills Development research programme of the HSRC

Further reading:

Bishop, A (1991) Mathematical Enculturation: A cultural perspective on mathematics education. Kluwer Academic Publishers, Dordrecht.

D’Ambrosio, U. (1989). A Research Program and a Course in the History of Mathematics: Ethnomathematics. Historia Mathematica, 16, 285 – 288.

Gerdes, P (1994) Reflections on ethnomathematics. For the Learning of  Mathematics 14(2): 19 – 22.

Gerdes, P. (1995): Women and Geometry in Southern Africa. Some suggestions for further research. Ethnomathematics Project. Universidade Pedagogica Mozambique.

Gerdes, P. (1999): Geometry from Africa: Mathematical and Educational Explorations. Mathematical Association of America.

Nkopodi N, and Mosimege M. D. (2009). Incorporating the Indigenous Game of Morabaraba in the Learning of Mathematics. South African Journal of Education, V29, 377-392